Gravity Constants,
October 14, 2014 Rev. Feb.
28, 2018
 ///
///
Home
Gravity Concepts
Create Momentum
Light Spd vs Relativity
Double Force Paradox >
Web--Site--Page--List
Gravitational Constants and Pressures
Abstract:
This is a public domain article.
- The Gravity Maximum, of our Solar System:....All planetary bodies larger than Earth exhibit the maximum gravity
of:
1.141 g, ....= 11.9 meters / sec^2, ....= 1.141 gram force / gram.
- The Gravitational Shielding Limit, Densare Max:....The gravitational shielding limit, Densare Max, is the minimum amount of matter
per unit area that will absorb all gravitational radiation. The gravitational shielding, Densare, for all planets is obtained by dividing
the planets apparent mass by the cross-sectional area.
- The Radiation Pressure Limit, A Solar System constant:....The
maximum potential radiation pressure for a planet is obtained by
multiplying the surface gravity by the shielding value, Densare Max,
which will stop all gravitational radiation
- Our Gravitational Constant, "G":.... is derived using only the radiation and
shielding concept and the features of our Solar system, it will
differ in other solar systems and will be proportional to their maximum
radiation pressure.
- Q. Majorana's Absorption Constant for Gravity:.... is reviewed
and replicated, using Solar system data. The resulting value of
gravitational absorption found by this study is approximately " 23 " times larger than that found by Dr. Majorana's experiments of circa
1920 AD.
- The Sun's true effective Mass:....can not exceed 4 % of NASA's Standard Model value. The
Sun's large magnetic field shields gravitational radiation making the Sun's mass
and Densare appear greater than actual. It is possible that the material
of the visible Sun is completely shielded by the central magnetic field and the
Sun does
not contribute to the solar central gravitational force. The measurable
gravitational shielding by the large magnetic fields demonstrate the causal unification of gravitational, inertial and electromagnetic forces via a
radiation and shielding force system.
- There are no gas planets:....The large planets consist of
a gravel mix with a density comparable to the density of the smaller planets.
Their "apparent" small
weight and inertia is attributed to the shielding of the bulk of the deep interior matter from
prime radiation. Prime radiation and shielding in this model are the cause of gravitation:
i.e. effective mass, weight and inertia.
***
GvR 1
The content in this article authored by me is granted to be
in the public domain, distribute and translate freely. Cheers.
Signed: Stanley V Byers 10/23/2014
The Gravity Maximum, a physical constant of our Solar System. All planets, larger
than Earth exhibit the maximum gravity
of 1.141 g, = 11.9 meters / sec^2, = 1.141 gram force / gram.
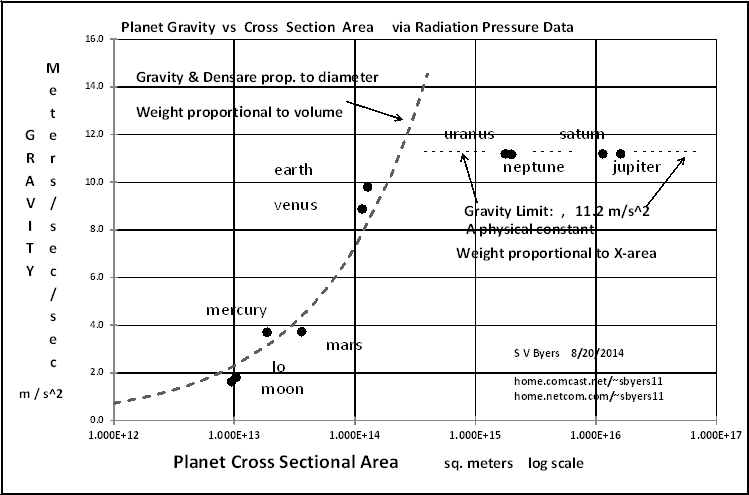
Figure 1
Gravity and Radiation Pressure Limits occur at Total Shielding. From Fig. 1
above, it is seen that radiation shielding and gravity are essentially
proportional to the diameter of small planets and moons. When
celestial objects become large enough,... all radiation is blocked and the
pressure and gravity become limited at the common maximum
value. All solar system objects larger than Earth exhibit total shielding and
therefore display the common maximum gravity of 1.141g,
or 11.9 m/sec^2. Continued
The common gravity limit of 1.141 g,...indicates that hypothetical
Black Holes and Neutron Stars can not exist in a radiation and shielding system.
Black Holes and Neutron Stars are hypothetical features of the Mass Attraction and General
Relativity attraction theories. The paper
"Force Doubling Paradox of Gravitational Attraction"
provides evidence showing that attraction models of gravitation are not realistic.
Saturn, the largest solar system object without an effective magnetic field shield, is
used as the gold standard for establishing and calibrating the constants:
- Gravity Maximum
- Densare Shielding Maximum
- Radiation Pressure Maximum
The surface gravity for Saturn is determined by the orbital force of it's
moons. The Orbital force of each moon indicates that Saturn's gravity is 1.141
g, = 1.141 gram force per gram. NASA's published value for Saturn's
gravity is 1.061 gram force per gram. ***
The gravitational shielding Densare for all planets is produced by dividing
the planets apparent mass by the cross-sectional area.
For the large black shadow planets the apparent weight, mass and inertia are
no longer proportional to volume but are exactly proportional to
the cross sectional area (Ax). The
numerical quantity of atoms is the only attribute that remains proportional to
volume.
From Fig.1 above the
common shielding Densare limit is evident for all planets larger than earth. The
maximum shielding value is 5.3366×10^9 grams per square centimeter.
In the original
DENSARE Graph Fig. 1 , Jupiter
is shown to have an anomalous shielding value approximately 3 times greater than
the maximum value for black shadow planets. However, the actual shielding of
Jupiter is the same as the other black shadow planets. The anomalous value is
due to the cross-sectional area of the large unseen magnetic field surrounding
Jupiter, which also shields gravitational radiation and thereby provides
additional apparent mass to Jupiter's position. ***
The maximum potential radiation pressure for a planet is obtained by
multiplying the surface gravity by the shielding value, Densare Max, which will
stop all gravitational radiation. This same method is used to find the reduced
pressure values for the smaller planets.
Saturn's radiation pressure equals the Radiation Pressure Limit, a constant
of our solar system. Given:
1g = 1 gram force / gram
Pr_sa = ( g_sa ) gf/gm x ( Dn_mx ) gm/cm^2
Pr_sa = 1.141 g x 5.3366 x 10^9 gm/cm^2
Pr_sa = Pr_mx = 6.09 x 10^6 kgf / cm^2
Pr_sa = 86.6 million PSI,
= Pr_max, = 600 GPa Approx.
The potential radiation pressure for each of the black shadow planets is
equal to this radiation pressure limit. This radiation pressure limit, Pr_max, a
constant derived in our solar system. ***
The Gravitational Constant, "G" is derived using only the radiation and
shielding concept and the known physical features of the Solar system as provided by NASA data.
This is not an attempt to improve or contest the accuracy of the published
conventional gravitational constants.
The goal is to demonstrate and validate a radiation and shielding system of
gravitation by correctly reproducing the standard gravitational constant using
the radiation pressure concept only, without mass attraction and GR attraction.
The Gravitational constant derived here, with the radiation pressure system of
gravity, matches NASA's derived value within 2 parts in 10,000 parts.
It will be derived by comparing the gravitational radiation flow and
pressure towards the Earth,...to the equal opposing orbital force of the Moon.
It is clear that the acceleration of the Moon toward the Earth is an effect
directly caused by radiation flow and potential pressure towards the Earth. The
Moon's opposing orbital centrifugal reaction force is used to gauge the
effective value of the Earth's potential pressure (gravity) at the Moon.
Since the gravitational constant is shown here to be
directly proportional to the maximum radiation pressure it has to be
considered a variable, that will depend on the
gravitational pressure within a particular solar system. This indicates that
our gravitational constant cannot be used for calculations with in other
solar systems.
The
Moon's
orbital force ( Fc = M * v^2 / r ) is found by using the Moon's mass
(M_mn), orbital velocity (v_mn) and the orbit's radius to the barycenter (yb_mn) as givens from
NASA. This orbital inertial reaction force is equal to and balances the
radiation force, ( g ) toward the
Earth.
This math derivation, using only
NASA's data and Mathcad 15, produces:
- The Orbital force of the Moon, Fc_mn , in Kgf
- The Earth's potential radiation press., Pr_e ,
in gf / cm^2 .
- The Earth's gravitational
force per gram, ( g ) in gf / gm, and
- The gravitational constant, ( G )
The Earth's gravity ( g ),
is derived without using Newton's equation, and
without using the gravitational constant ( G ).
Ref.:
Addendum C: Math Assumptions
|
Deriving a Gravitational Constant
Givens: NASA data for Earth's Moon |
| One g = one gram force / gram mass |
1 g = 1gf / gm |
| Mass of Moon, M_mn
|
 |
| Projected Area of Moon, Effective |
 |
| Orbital Velocity, v_mn |
 |
| Radius to barycenter,
yb_mn |
 |
| Distance between Earth / Moon centers |
 |
| Moon's Shadow density,
Densare, Dn_mn |
 |
| One "g" per NASA |
 |
| Shadow density value ( Dn_max ) which
stops all
Gravitational Radiation,...a physical constant in our
solar system. |
 |
Earth's: Mass
Radius |
M_e = 5.9736 x 10^24 kg
r_e = 6.3761 x 10^6 meters |
Q. Majorana's Absorption Constant for Gravity is reviewed and replicated, using
Solar System data, and a radiation and shielding model of gravity. The resulting value of
the gravitational absorption ratio found by this
study is approximately
23 times larger than the value found by Dr. Majorana's experiments of circa
1920 AD.
Q. Majorana's excellent vision and shielding work of circa 1920 AD, was first and he was first to
publish and was correct in
demonstrating that gravitational shielding exists. Except for one small error it
appears that he
would have found the same larger shielding rate that is now evidenced by this
work via a radiation and shielding system of gravity.
Majorana apparently assumed that gravity could be due to:
1) external radiation pressure, " or " 2) attraction.
As a result, he provided shielding both above and
below the test mass on all tests.
Current absorption studies using a radiation and shielding system indicate
that if Majorana had placed a shield only above the test mass, he could have
found the absorption ratio to be 23 times larger than his published data.
In this radiation and shielding model, the coherency and the shielding of the
prime radiation are the main causes of
gravity. When the coherent radiation encounters and penetrates the planet it is not
absorbed, it is reflected from the internal atoms and nucleons and scattered. The gravitational force decreases with depth
not because of absorption, but because the effective coherent radiation is
scattered via reflection into ineffective in-coherent radiation. The reflected
radiation is obviously spatially incoherent, since it is diverging from a
multitude of
point sources.
Majorana's absorption constant can also be called Majorana's shielding rate.
Gravitation's reduction with depth follows an exponential curve because the
loss is proportional to the reduced radiation value at depth. Intuition
erroneously suggests that the absorption constant would remain constant for all
planet sizes. However, planet data reveals that the absorption constant is not
constant, it increases by approximately 20% for Earth and Venus in relation to
the moon and small planets, Fig. 2. All of the large black shadow planets
have the same maximum absorption rate which is 47 % greater than the rate for
the small moons and planets. A portion of the reflected radiation apparently
interferes with the incoming radiation force and thus increases the static shielding rate.
This reflection feature accounts for the linear increase of the absorption ratio with planet size.
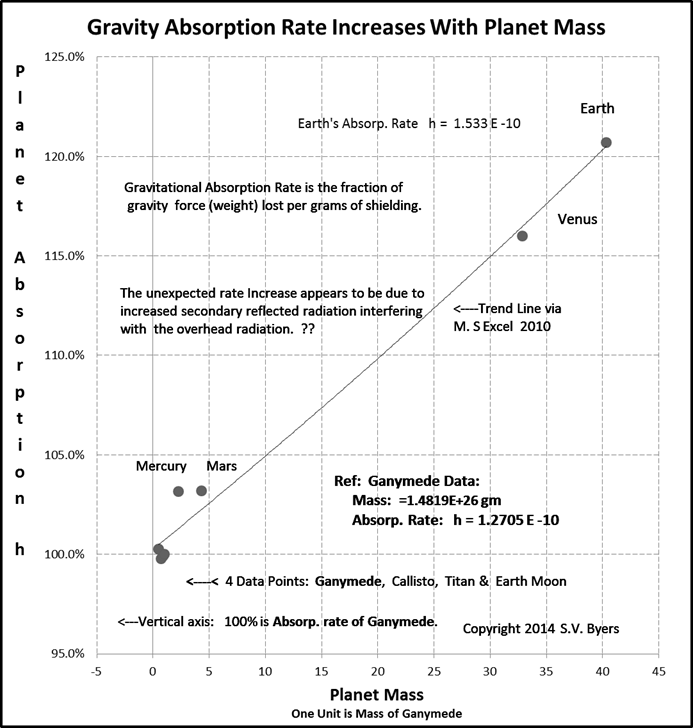
Gravitational Absorption vs Planet Mass
Figure 2
In this model the incoherent reflected prime radiation becomes coherent again
as it completes its cycle by returning to merge with the coherent radiation of
outer space. A closed cycle removes the concern that the prime radiation may be
consumed through absorption.
Continued
Gravitational absorption via Exponential Decay
Ref. Givens above, via Math Cad 15.
|
|
Earth's Rad. Press. with Depth, Pr_i.e |
 |
| For the earth and small planets the
surface pressure and the exit pressure are readily known. With two known
points on the exponential decay profile, the curve and the absorption
rate are readily obtained.
|
| Dividing through by Pr_max and taking
the natural log of each side provides the equation for obtaining the absorption
ratio per depth, 1/(cm) |
 |
| Earth's Average Absorp. ratio per depth. Lm.e 1/cm |
 |
| Earth's Absorp. ratio, h.e
in gram loss per gram / cm^2 will be derived in the next section. |
Earth's avg. effective density and Earth's absorption ratio
per gram via the radiation and shielding system.
The exponential equation work given above only produces an absorption rate
per depth. Majorana's name for the per depth rate is lambda, the symbol used is
Lm.
In order to obtain the absorption rate per gram from the Lambda rate, the
effective density of the material must be found. Majorana knew the density of
his shielding material, but the Earth's average effective density for a radiation
shielding system is unknown and may be found with the following mathematics.
| Absorption Rate per Gram: h.e
Ref: Givens provided above |
The "Layer Cake" formula for Earth Mass.
Ref: Wikipedia disk integration info:
https://en.wikipedia.org/wiki/Disk_integration |
 |
Integrating these above terms over the
diameter
of the Earth gives the volume. |
 |
| These terms provide the proper "density" for each layer of integration.
Assuming a homogenous mix of atoms. |
 |
The only unknown in the M.e equation is
effective density, rho.e. Increasing rho.e to 10.69 gm/cm^3 provides the
Earth's known mass of:
Earth, NASA given: M.e = 5.9736 x 10 ^24 kg |
M.e = 5.9736 x 10 ^24 kg
therefore
rho.e = 10.69 gm/cm^3 |
Inserting rho.e into the Lamda equation provides
Earth's
absorption ratio per gram , h.e |
h.e = Lm.e / rho.e |
h.e = 1.53 x 10^-10
2014 AD |
Q Majorana's Absorption Rate,
h.qm
1920 AD vs 2014 |

1920 AD |
h.e / h.qm = 23 |
Using Dr. Q. Majorana's equations, a
radiation and shielding system and NASA's data,... Majorana's absorption rate has
been updated to: h = 1.53 x 10E(-10) . ***
////////////////////////
Within a radiation and shadowing system of gravity, a free body in free
space is bathed by an equal amount of radiation force from all sides, which
gives an isotropic force balance condition. As a free body ventures closer
to a planet it would be shielded from a portion of the radiation from the
direction of the planet. Since the free space balanced radiation force is
now unbalanced, an acceleration toward the planet will result. Once in a
stable orbit the body's outward centrifugal inertial force balances the
radiation pressure towards the planet's position.
In studies of the past it was assumed that gravitational
force arose as a physical action (attraction) that occurred only in the space between the
two bodies of mass. With that assumption it seemed reasonable that a shield
between the two bodies would decrease the gravitational force.
However, within the geometry and physical mechanics of a radiation and
shadowing system it is obvious that an additional shield beneath a test mass
will increase the
measured weight of the
test mass by adding to the density of the planets gravity shadow. This
increase of force is clearly demonstrated by the measurements taken for two
gravimeter calibration test programs of the 1990's.
The following quote is a short description of the calibration method used
by the 1994 and 1997 calibration tests preformed by G. Casula, et al.
Quote In
our station a new calibration method is used: it consists in moving a
mass of simple geometry ( circular ring with square cross section ), along
the vertical axis of the instrument, in order to perturb ( Shield )
the gravity field in a known way, ( Achilli et al., 1995; Varga et al,.
1995 ) Unquote
False Data using "Mass Attraction"
It is possible to generate the theoretical affect curve in the following chart using Newton's equation and the mass attraction concept. However; The paper "Force Doubling Paradox of Gravitational Attraction" adequately demonstrates that mass attraction is not a realistic concept.
Figure 3, below, is a chart
of the calibration measurements
using the circular ring method. (Chart's notes are by current author.)

Figure 3
Calibration Signal for Superconducting Gravimeter
GWR, 1997 from
http://www.earth-prints.org/bitstream/2122/1569/1/12%20casula.pdf .
This particular calibration chart, Fig. 3, was selected because it presents
the complete data curve for the vertical motion of the ring calibration
mass. It is clear that when the shielding ring mass is above
the gravimeter sensor the force of gravity is reduced,...and
when the ring mass is lowered below the sensor the apparent
weight force is increased.
This indicates that if identical rings were placed in the above and below shielding positions at
the same time, the lower ring would mask a very large percentage of the
shielding due to the above ring,...and therefore, very little or no shielding
action would be found.
This is essentially the problem that Dr. Q. Majorana encountered when he
shielded the above and below positions at the same time for his 1920 shielding
tests. His delta g shielding test should have resulted in a reading of at least
30 microGals, instead of the recorded low value of
~ 1 microGal.
It is seen that when the ring is above the sensor the calibration test is
successfully achieving the intended goals of Dr. Q. Majorana's shielding project
of 1920. A replication of Majorana's experiment should be conducted without the
shielding below the test mass. It will be necessary for any new Majorana
replication experiments to follow the ring test procedure and make separate
measurements for shielding above and below the test mass (gravimeter sensor).
Two gravimeter shielding calibration tests by others are: Varga
1994 and
Casula 1997.
The original purpose of these ring calibration projects was to
provide a very accurate calibration for these gravimeters. One does not
necessarily have to know the true first cause of gravity in order to perform an
accurate calibration of a gravimeter. However;...this ring calibration method
and the resulting data are repeatable, valuable and clear evidence that the cause of gravity is a radiation and shadowing system.
***
The radiation and shielding system and math presented here indicate that:
- The material Sun contributes 4 percent or less of the
mass and
gravitational force displayed by the center of the
Solar system.
- Jupiter contributes only 43 percent or less of
the mass and gravitational force for its location.
The individual magnetic fields surrounding the Sun and Jupiter shield the
gravitational radiation in the same manner as solid matter and this explains
the bodies "apparent" anomalous excess mass.
All planets and Suns larger than Earth provide total
shielding of gravitational radiation. In this work these large planets are
called black shadow planets, since their projected shadow contains no radiation
from the direction through the planet. These large planets, except Jupiter, all have the same
maximum potential radiation pressure of 86.6 million
PSI at the surface, and all have the same surface gravity of 1.141 g.
In order for the Solar system center to provide enough gravitational force to
maintain the planets in orbit, Nasa data indicates that the Sun's surface
gravity must be 27.96 g. Since the maximum surface gravity in our radiation
and shielding system is 1.141 g, the cross-sectional area of the Sun
would have to be 24.5 times larger than it's visual cross-sectional area in order
to produce the required force.
The magnetic field of the Solar system center shields gravity in the same manner
as solid matter and provides the large area of shielding required for the total
force towards the solar system center. This gravitational shielding via a magnetic
field produces a true gravitational force that is not related to magnetic "attraction".
If the gravitational shadow of the magnetic field is a black shadow shield, it's
projected area must be 24.5 times larger than the visual Sun, with a total diameter five
times that of the Sun. If it is a gray shadow shield the area must increase
accordingly.
Since the magnetic shielding surrounds the spherical visual Sun, the Sun
itself may exist within a field of very low gravity and therefore have a surface
gravity approaching zero. With low gravity, inertia and pressure at the surface of
the visible Sun, the large looping mass ejections seem predictable and may be
due to meteor impacts and their molecular disintegration. Since the Sun appears
to have no atmosphere or atmospheric pressure, meteors would arrive at the visual surface intact after
traveling through the magnetic gravitational shield. Energetic disintegration of
the meteor may occur as it enters the area of zero gravitational pressure and
near zero mechanical pressure.
If the Sun has near zero gravity at its visual surface, a zero gravity core
boundary may exist below, near the surface and encompass most of the Sun's material.
However, if the visual Sun is a complete plasma it may not have enough
gravitational shielding to produce a zero gravity core.
The material within all zero gravity cores is expected to be a nucleon and atom plasma
within a field of incoherent prime radiation. Incoherent radiation produces no
gravitational force. If the surface gravity of the Sun is at one percent of the
maximum, the internal mechanical pressure must also be only one percent of the
maximum gravitational radiation potential pressure of our Solar System as listed in the next paragraph.
Unlike the black shadow Sun and Jupiter, the other black shadow planets have maximum
gravity at their surface and a large zero gravity core. The zero gravity core
exists because all gravitational radiation is absorbed before reaching the
planet center. This core would support the mechanical weight pressure of the
outer layers and the atmosphere, but the core material would not exhibit weight or inertia.
The isotropic mechanical pressure within the large planets zero gravity cores would equal the
maximum gravitational radiation potential pressure of the our Solar System, 86.6 million
PSI, [ 6.09 meg Kgf/cm^2 , 587.1 GPa ] . Theoretically, there will
be no gradient of the mechanical pressure within the boundary of this zero gravity core.
Jupiter, a black shadow planet, is the only planet in our solar system that
exists within a magnetic field which is large enough to shield gravitational
radiation. NASA data predicts that Jupiter's surface gravity must be 2.54 g in
order to maintain it's moons in their present orbits. Since the maximum surface
gravity in our Solar System is 1.141 g, the cross-sectional area of Jupiter would
have to be 2.23 times larger than it's visual cross-sectional area in order to
produce the required force.
The existence of Jupiter's atmosphere demonstrates that unlike the Sun,
Jupiter has some surface gravity. This indicates that the magnetic field only
provides partial shielding. Therefore the body of Jupiter will provide a black
shadow area in the center of the magnetic gray shadow area. The magnetic field
then provides an extended gray shadow area sufficient to produce the total
required force.
The web article on this site,
Magnetism as Radiant Whirlpools , presents
the concept of magnetism as a soft unstable form of matter.
Current literature and NASA continue to refer to the large planets as gas planets.
The persistent scars left on Jupiter by the Shoemaker Levy comets is evidence
that it is not a ball of gas. A radiation and shadowing system predicts that the
large planets have a surface just as solid as the small planets. The apparent
low density exists because a large portion of the mass of each large planet is internally
shielded from gravitational and inertial radiation.
A review and understanding of the mechanics of a radiation and shielding
system of gravity will aid in studying the Sun and Jupiter shielding and the mass
anomalies presented here.
The radiation and shielding system of gravity is presented in the paper, "Radiant Pressure Model of Remote Forces",
available at the URL
https://energy-gravity.comgrav11.htm on this web site.
The rate, d(D)/dy, of decrease of gravitational force (D) due to shielding by material depth (y) is:
Eq. 3 d(D) / dy = (-) Lm x D
or (1 / D) x d(D) = ( - )Lm x dy
By separation of variables and integrating both sides we obtain the natural Log Equation 3a where C is a constant of integration.

Eq. 3a Logn (D) = (- Lm x y )+ C1
Natural log base e
Solving for D we obtain Equation 3b:
Eq. 3b D = C2 x e^(-Lm x y)
On the surface (y) is equal to zero, therefore the constant C2 is equal to
Do. So Equation 4 results.
Eq. 4 D = Do x e^(-Lm x y)
Solving for Lm
Eq. 5 ln(D/Do) = -(Lm x y)
Eq. 6 Lm = - ln(D/Do) / y
***
( I ) Capital I is the Light intensity,
a variable that decreases with depth below the surface.
( Io ) is the Light Intensity, a constant at the surface.
( Lm ) is the absorption ratio and is determined by the the
clarity of the water.
( y ) is the depth in meters of water shielding the light.
( e ) is the base for natural logarithms.
The loss of intensity at any given depth in meters
(y) is proportional to the reduced intensity (I) at that depth. This is shown in
Equation 3. Lm
is the absorption ratio and its average value depends on the clarity of the
water.
Equation 3
d(I)/dy = (-) Lm x I
This second equation shows that the light intensity , (I) decays as a
natural exponential function with the depth, (y), of shielding .
Equation 4
I = Io x e^( - Lm x y )
For the clear lakes of Northern Michigan the shielding rate (Lm) is 0.15068 when the depth is measured in
meters. This value of shielding reduces the light to 50 percent at a depth of 4.6
meters, (15 ft.). At a depth of 30.56 meters, (99 ft.), the light has been
reduced to one percent . The math steps for obtaining Eq. 4 from Eq. 3 is
provided in Addendum A , above. ***
In order to mathematically model the forces of the solar system, the
following simplifying assumptions are made for the system's unknown physical properties.
- A homogeneous distribution of matter exists in each system body.
- The average maximum "effective" mass density occurs at the surface due to shielding of
the interior.
- The effective mass density, inertial and weight of all system bodies decreases with depth due to
shielding.
- All planets larger than Earth have a large inner core with zero gravity,
mass
and inertia, due to complete shielding by the upper layers.
- There are no known gas planets.
- For small planets a zero gravity point exists at the center due to a
balance of radiation from opposing sides.
- The gravitational absorption rate increases with planet size due to backscatter of radiation.
- The physical constants found for our solar system may not apply to our Universe.
- The galactic magnetic field encompassing our system appears to shield and reduce
our maximum gravitational pressure to a value below the maximum of the
galaxy.
- There are no attractive forces and no action at a distance forces within
this radiation shielding system of gravitation, electrostatics, magnetism
and cohesion of particulate and nucleons.
- A planets physical properties of weight, mass, density, inertia and
moment of inertia, are all subject to the effects of shielding.
- Gravity is a force per unit mass and the dimensional units of
grams force per gram mass will be the basic units used
for this study of gravity,...i.e.,... 1g = 1gf / gm
.
Using 1g as an acceleration will be avoided in the
calculations for this radiant force model. ***
 ///
///



























